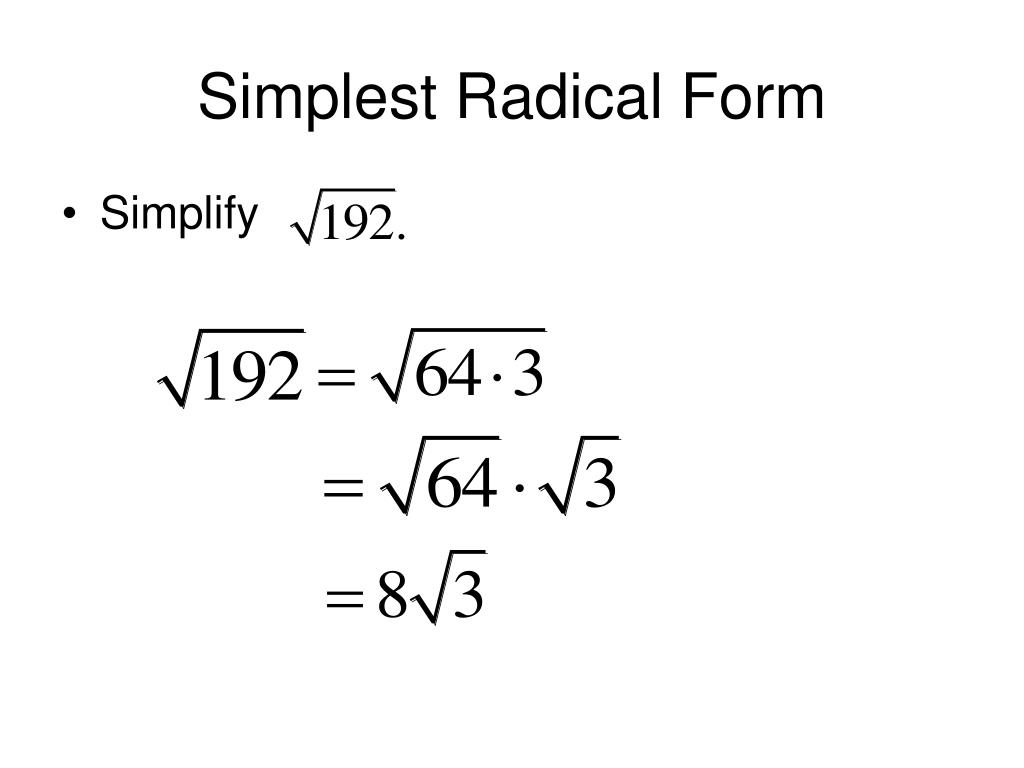Pythagorean Theorem Radical Form
Pythagorean Theorem Radical Form - Estrada 691 subscribers subscribe 14k views 6 years ago basic video in which i demonstrate how to simplify. That is, leg2 + leg2 = hypotenuse2. Find the length of the hypotenuse. X 6 11 7 8 solution: Web 0:00 / 12:15 the pythagorean theorem and simplest radical form mrmaisonet 52.9k subscribers subscribe 0 share no views 55 seconds ago practice finding the missing. The pythagorean theorem is a cornerstone of math that helps us find the missing side length of a right triangle. We can find the total area of the square by squaring any one of the sides of the square. The hypotenuse is the longest side, opposite the right angle. The first topic of this video models how to write square root expressions in simplest radical form. Identify the legs and the hypotenuse of the right triangle.
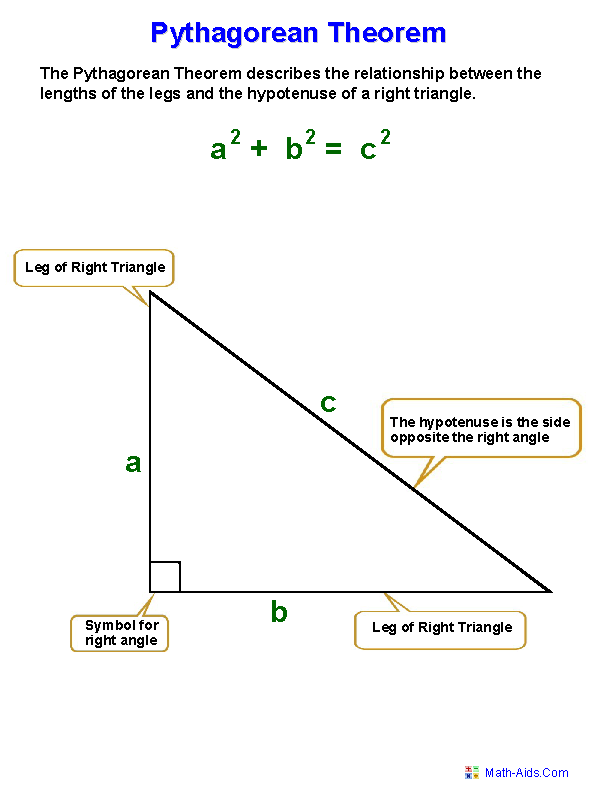
Identify the legs and the hypotenuse of the right triangle. Find the length of the hypotenuse. Web hence, they must be equal to one another. Web one of the best known mathematical formulas is pythagorean theorem, which provides us with the relationship between the sides in a right triangle. Web pythagorean theorem simplest radical form 75 results sort: The hypotenuse is the longest side, opposite the right angle. Web this calculator solves the pythagorean theorem equation for sides a or b, or the hypotenuse c. Web proof of the pythagorean theorem. Find the missing side in simplest radical form. A and b are the legs.
This includes calculating the hypotenuse. X + 4 + 16 = x. So this is 2 times 216. Get them up and moving with this. Second, this video discusses and uses the. Web hence, they must be equal to one another. Find the unknown leg in the right triangle, in simplest radical form. Web simplest radical form and the pythagorean theorem. In this tutorial, you'll get introduced to the pythagorean theorem and see how it's used to solve for a missing length on a right triangle! The lengths of the legs are 6 and 8, and the length of the hypotenuse is x, so 6 2 + 8 2 = x
PPT Simplifying Radicals & Pythagorean Theorem PowerPoint
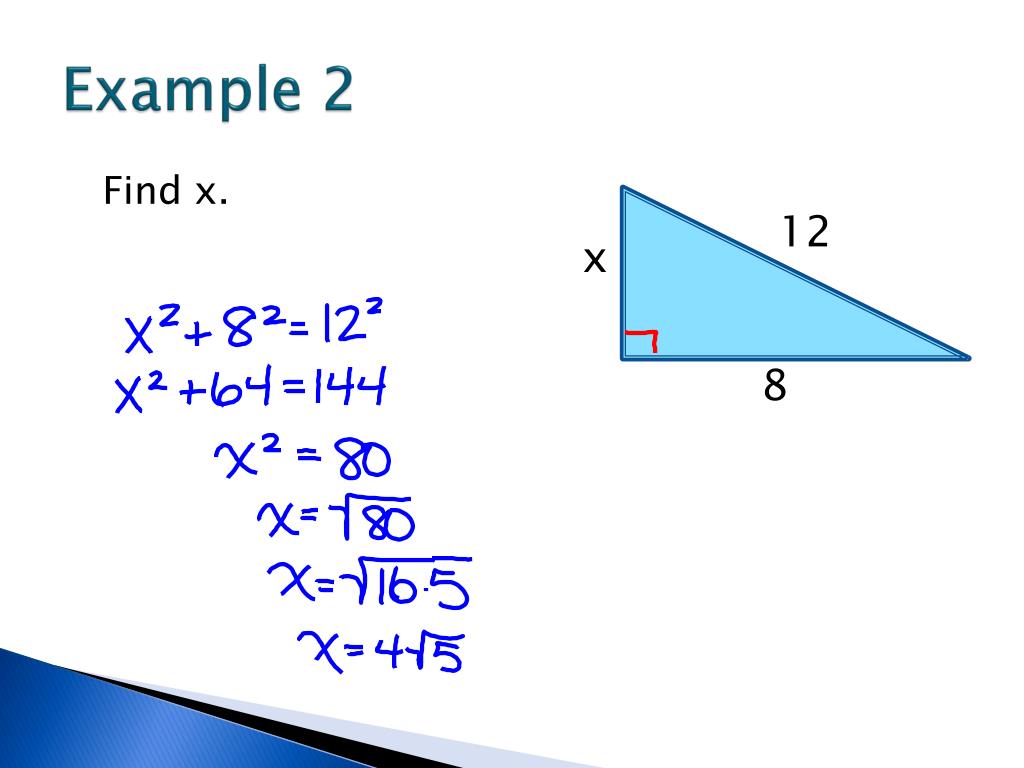
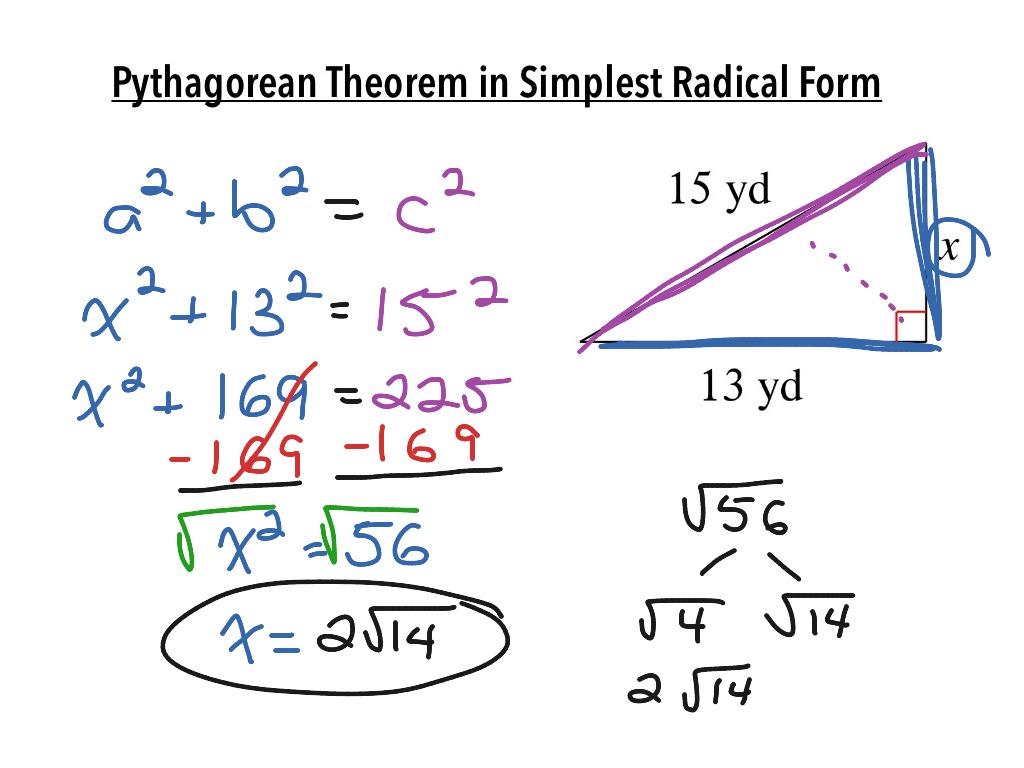
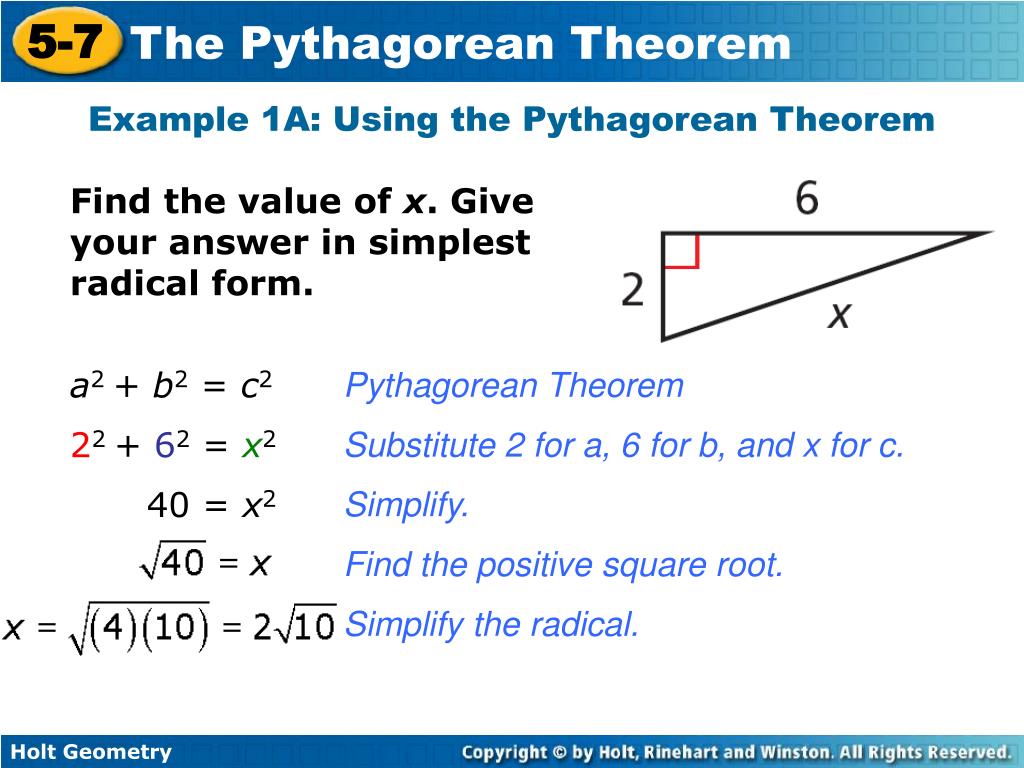
Web write all answers in simplest radical form. That is, leg2 + leg2 = hypotenuse2. Find the unknown leg in the right triangle, in simplest radical form. Web hence, they must be equal to one another. Web the pythagorean theorem states that the square of hypotenuse is equal to the sum of the squares of the other two sides.
Pythagorean Theorem in Simplest Radical Form Math, Algebra
Web the pythagorean theorem is a very popular theorem that shows a special relationship between the sides of a right triangle. Web this pythagorean theorem calculator will calculate the length of any of the missing sides of a right triangle, provided you know the lengths of its other two sides. A2 + b2 = c2 subtract 2ab from both sides..
Pythagorean Theorem Formula Images & Pictures Becuo
A and b are the legs. Web pythagorean theorem simplest radical form 75 results sort: Web 0:00 / 12:15 the pythagorean theorem and simplest radical form mrmaisonet 52.9k subscribers subscribe 0 share no views 55 seconds ago practice finding the missing. The lengths of the legs are 6 and 8, and the length of the hypotenuse is x, so 6.
PPT Use the Pythagorean Theorem and its converse to solve problems
A right triangle consists of two legs and a hypotenuse. Find the missing side in simplest radical form. The pythagorean theorem formula is: Web pythagorean theorem radical form 266 results sort: Find the unknown leg in the right triangle, in simplest radical form.
PPT Simplifying Radicals & Pythagorean Theorem PowerPoint
The legs have length 24 and x are the legs. Web use the pythagorean theorem to determine the length of x. Pythagorean theorem with radicals error analysis google forms and printable by absolute algebra 4.7 (11) $4.00 pdf google apps™ X 6 11 7 8 solution: A and b are the legs.
PPT The Pythagorean Theorem PowerPoint Presentation, free download
Find the unknown leg in the right triangle, in simplest radical form. It can provide the calculation steps, area, perimeter, height, and angles. That is, leg2 + leg2 = hypotenuse2. Find the missing side in simplest radical form. Web pythagorean theorem calculator to find out the unknown length of a right triangle.
PPT 8.1 Pythagorean Theorem and Its Converse PowerPoint Presentation
Find the unknown leg in the right triangle, in simplest radical form. Web simplest radical form and the pythagorean theorem. In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the legs. Find the length of the missing side. A2 + b2 = c2 subtract 2ab from both sides.
pythagorean theorem * solve for x ( leave response in simplest radical
Web write all answers in simplest radical form. In this tutorial, you'll get introduced to the pythagorean theorem and see how it's used to solve for a missing length on a right triangle! In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the legs. X 6 11 7 8 solution: Find.
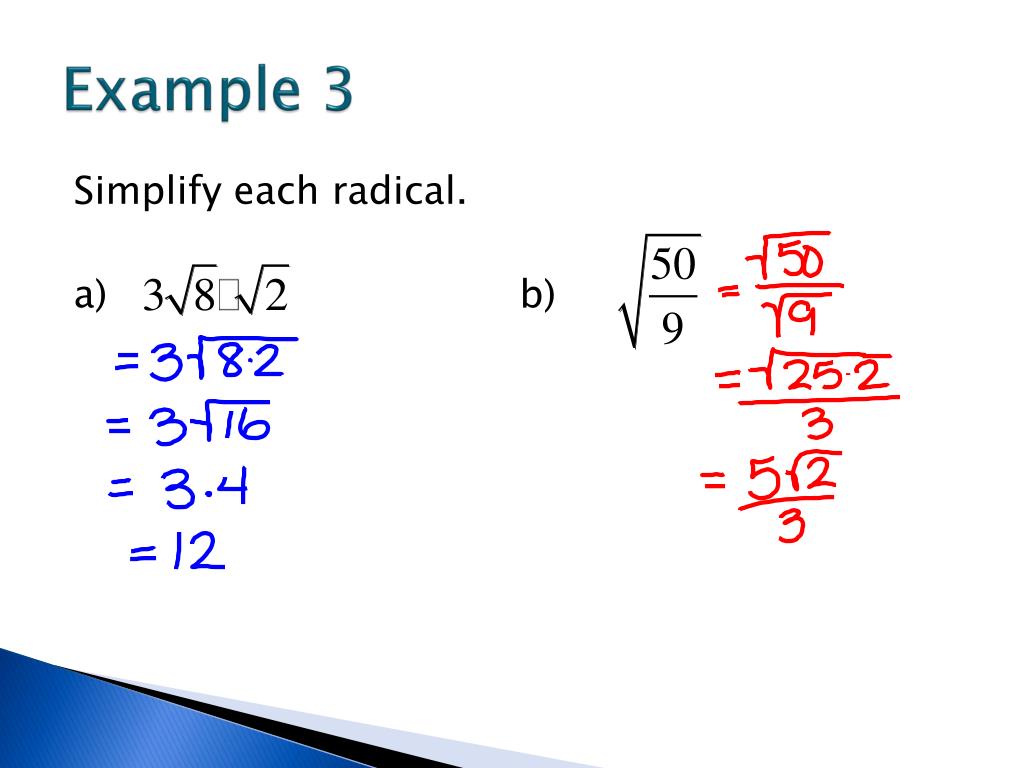
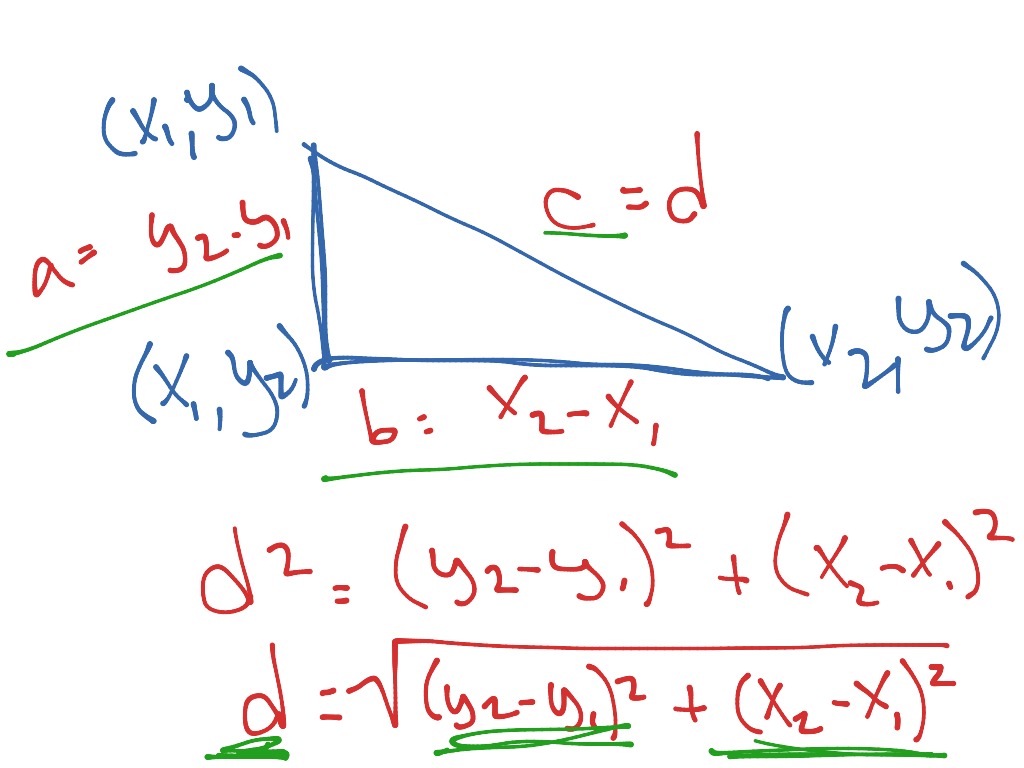
Simplest Radical Form/Pythagorean Theorem/Distance Formula Math ShowMe
X + 4 + 16 = x. Web simplest radical form and the pythagorean theorem. A right triangle consists of two legs and a hypotenuse. In this tutorial, you'll get introduced to the pythagorean theorem and see how it's used to solve for a missing length on a right triangle! The last equation, a2 + b2 = c2, is called.
Pythagorean Theorem with Radicals YouTube
We can find the total area of the square by squaring any one of the sides of the square. In a right triangle, the square of the hypotenuse is equal to the sum of the squares of the legs. A) (b) 6 x 10 x 4 √ 2 answers ii. Find the length of the missing side. A 2 +.
Web Write All Answers In Simplest Radical Form.
A) (b) 6 x 10 x 4 √ 2 answers ii. Second, this video discusses and uses the. Web hence, they must be equal to one another. Pythagorean theorem with radicals error analysis google forms and printable by absolute algebra 4.7 (11) $4.00 pdf google apps™
Web This Calculator Solves The Pythagorean Theorem Equation For Sides A Or B, Or The Hypotenuse C.
Web pythagorean theorem calculator to find out the unknown length of a right triangle. Web one of the best known mathematical formulas is pythagorean theorem, which provides us with the relationship between the sides in a right triangle. Web pythagorean theorem legs example 1: Web the pythagorean theorem states that the square of hypotenuse is equal to the sum of the squares of the other two sides.
Find The Unknown Leg In The Right Triangle, In Simplest Radical Form.
Web proof of the pythagorean theorem. 22 +x2 =62 ⇒ x = p 62 − 22 = √ 32 =4 √ 2 √ ab = √ a √ b exercise 1. Web we saw what the answer is, but maybe we can write it in kind of a simplified radical form. Web introduction pythagorean theorem and simplifying radicals mr.
In A Right Triangle, The Square Of The Hypotenuse Is Equal To The Sum Of The Squares Of The Legs.
X 6 11 7 8 solution: The hypotenuse is the longest side, opposite the right angle. See the solution with steps using the pythagorean theorem formula. Find x in the following: