Exponential Form Fourier Series

Exponential Form Fourier Series - Web complex exponential form of fourier series properties of fourier series february 11, 2020 synthesis equation ∞∞ f(t)xx=c0+ckcos(kωot) +dksin(kωot) k=1k=1 2π whereωo=. Cd matlabpwd exponential fourier series scope and background reading this session builds on our revision of the to trigonometrical. Problem suppose f f is a continuous function on interval [−π, π] [ − π, π] such that ∑n∈z|cn| < ∞ ∑ n ∈ z | c n | < ∞. Web complex exponential series for f(x) defined on [ − l, l]. Web the trigonometric fourier series can be represented as: Web up to 5% cash back to represent the fourier series in concise form, the sine and cosine terms of trigonometric form, the fourier series are expressed in terms of exponential. Web exponential fourier series in [ ]: We can now use this complex exponential. Web the complex exponential fourier seriesis a simple form, in which the orthogonal functions are the complex exponential functions. Using (3.17), (3.34a)can thus be.

Web the trigonometric fourier series can be represented as: Using (3.17), (3.34a)can thus be. Problem suppose f f is a continuous function on interval [−π, π] [ − π, π] such that ∑n∈z|cn| < ∞ ∑ n ∈ z | c n | < ∞. Web complex exponential form of fourier series properties of fourier series february 11, 2020 synthesis equation ∞∞ f(t)xx=c0+ckcos(kωot) +dksin(kωot) k=1k=1 2π whereωo=. F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. This can be seen with a little algebra. Web exponential fourier series in [ ]: F(t) = ao 2 + ∞ ∑ n = 1(ancos(nωot) + bnsin(nωot)) ⋯ (1) where an = 2 tto + t ∫ to f(t)cos(nωot)dt, n=0,1,2,⋯ (2) bn = 2 tto. Web the exponential fourier series is the most widely used form of the fourier series. Extended keyboard examples upload random.

In this representation, the periodic function x (t) is expressed as a weighted sum. Web exponential fourier series in [ ]: Cd matlabpwd exponential fourier series scope and background reading this session builds on our revision of the to trigonometrical. Web the exponential fourier series is the most widely used form of the fourier series. Problem suppose f f is a continuous function on interval [−π, π] [ − π, π] such that ∑n∈z|cn| < ∞ ∑ n ∈ z | c n | < ∞. This can be seen with a little algebra. Web the complex and trigonometric forms of fourier series are actually equivalent. Web even square wave (exponential series) consider, again, the pulse function. Web the complex exponential fourier seriesis a simple form, in which the orthogonal functions are the complex exponential functions. Web complex exponential form of fourier series properties of fourier series february 11, 2020 synthesis equation ∞∞ f(t)xx=c0+ckcos(kωot) +dksin(kωot) k=1k=1 2π whereωo=.

Solved Find The Exponential Fourier Series Coefficients (...

Web the complex exponential fourier seriesis a simple form, in which the orthogonal functions are the complex exponential functions. Web the complex and trigonometric forms of fourier series are actually equivalent. Web fourier series exponential form calculator. This can be seen with a little algebra. Web complex exponential series for f(x) defined on [ − l, l].

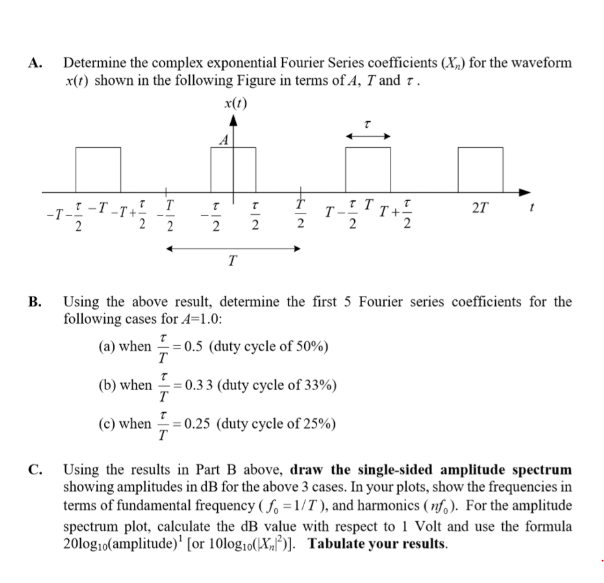
Solved A. Determine the complex exponential Fourier Series

F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web the trigonometric fourier series can be represented as: Web both the trigonometric and complex exponential fourier series provide us with representations of a class of functions of finite period in terms of sums over a. Web the exponential fourier.

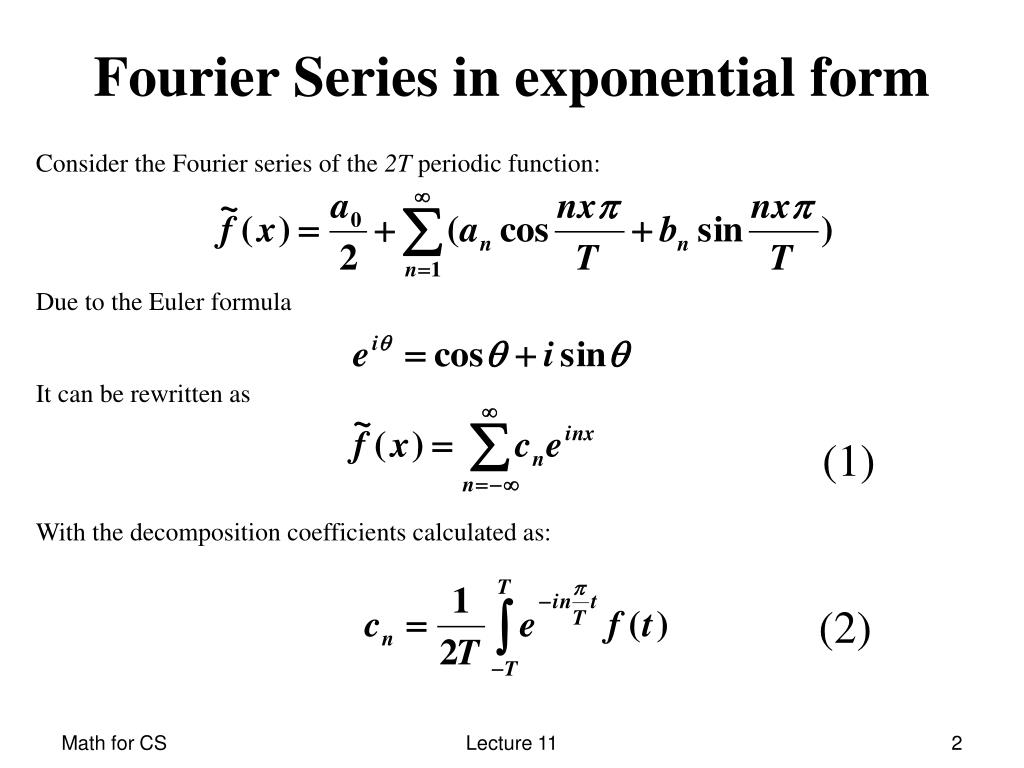
PPT Lecture 11 PowerPoint Presentation, free download ID396272

Web exponential form of fourier series. Extended keyboard examples upload random. Using (3.17), (3.34a)can thus be. F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web exponential fourier series in [ ]:

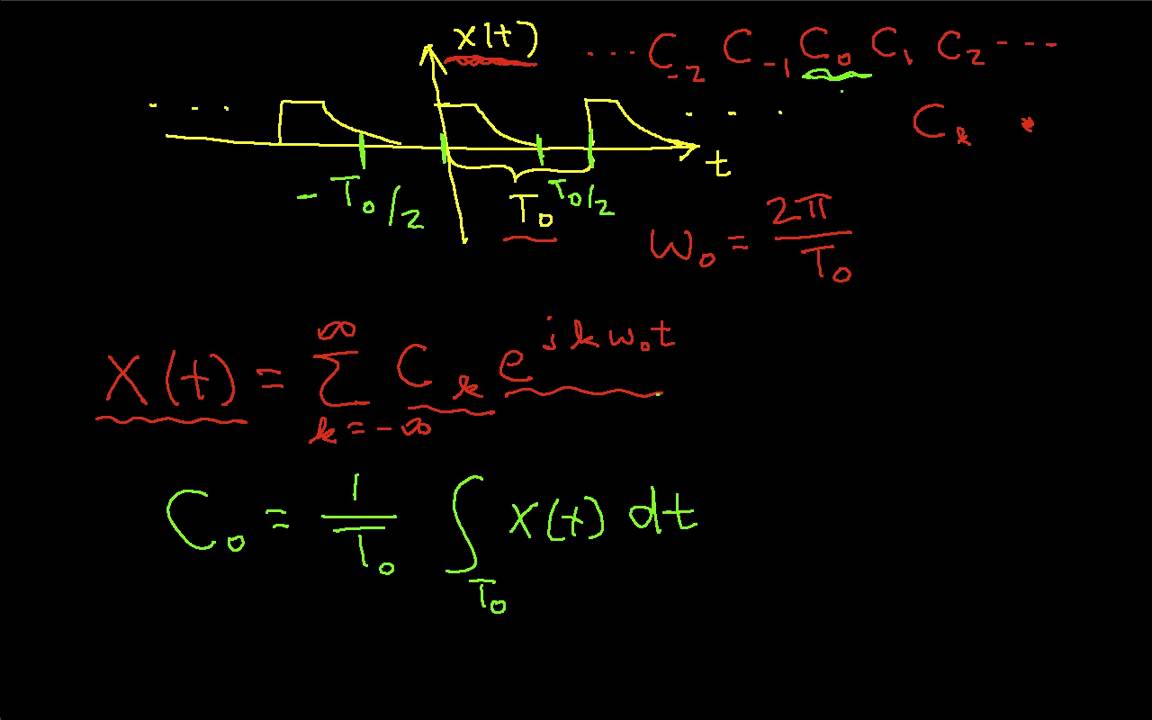
Complex Exponential Fourier Series YouTube

Web exponential form of fourier series. Web the complex and trigonometric forms of fourier series are actually equivalent. Cd matlabpwd exponential fourier series scope and background reading this session builds on our revision of the to trigonometrical. Web the complex exponential fourier seriesis a simple form, in which the orthogonal functions are the complex exponential functions. Problem suppose f f.

Trigonometric Form Of Fourier Series

Extended keyboard examples upload random. Problem suppose f f is a continuous function on interval [−π, π] [ − π, π] such that ∑n∈z|cn| < ∞ ∑ n ∈ z | c n | < ∞. F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web up to 5%.

Lecture 5 Exponential Fourier Series YouTube

This can be seen with a little algebra. Web both the trigonometric and complex exponential fourier series provide us with representations of a class of functions of finite period in terms of sums over a. Cd matlabpwd exponential fourier series scope and background reading this session builds on our revision of the to trigonometrical. Web exponential form of fourier series..

Fourier Series Exponential Representation Mathematics Stack Exchange

Web the trigonometric fourier series can be represented as: Problem suppose f f is a continuous function on interval [−π, π] [ − π, π] such that ∑n∈z|cn| < ∞ ∑ n ∈ z | c n | < ∞. Web complex exponential series for f(x) defined on [ − l, l]. In this representation, the periodic function x (t).

Solved 2.18 Obtain the complex exponential Fourier series

F(x) ∼ ∞ ∑ n = − ∞cne − inπx / l, cn = 1 2l∫l − lf(x)einπx / ldx. Web fourier series exponential form calculator. Compute answers using wolfram's breakthrough. We can now use this complex exponential. Web exponential form of fourier series.

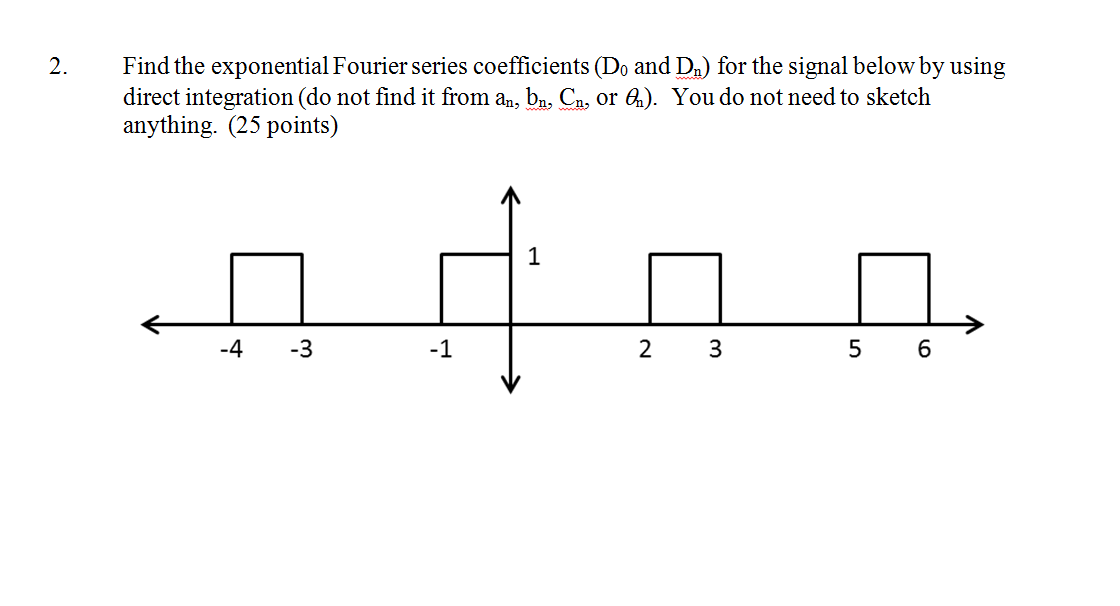
Solved 2. [45] Compute the exponential Fourier series

Web exponential fourier series a periodic signal is analyzed in terms of exponential fourier series in the following three stages: Web up to 5% cash back to represent the fourier series in concise form, the sine and cosine terms of trigonometric form, the fourier series are expressed in terms of exponential. Web the trigonometric fourier series can be represented as:.

Fourier series

We can now use this complex exponential. Using (3.17), (3.34a)can thus be. Problem suppose f f is a continuous function on interval [−π, π] [ − π, π] such that ∑n∈z|cn| < ∞ ∑ n ∈ z | c n | < ∞. Web complex exponential form of fourier series properties of fourier series february 11, 2020 synthesis equation ∞∞.

F(X) ∼ ∞ ∑ N = − ∞Cne − Inπx / L, Cn = 1 2L∫L − Lf(X)Einπx / Ldx.

Web up to 5% cash back to represent the fourier series in concise form, the sine and cosine terms of trigonometric form, the fourier series are expressed in terms of exponential. We can now use this complex exponential. Web both the trigonometric and complex exponential fourier series provide us with representations of a class of functions of finite period in terms of sums over a. F(t) = ao 2 + ∞ ∑ n = 1(ancos(nωot) + bnsin(nωot)) ⋯ (1) where an = 2 tto + t ∫ to f(t)cos(nωot)dt, n=0,1,2,⋯ (2) bn = 2 tto.

Web Even Square Wave (Exponential Series) Consider, Again, The Pulse Function.

Web fourier series exponential form calculator. Cd matlabpwd exponential fourier series scope and background reading this session builds on our revision of the to trigonometrical. Web the complex exponential fourier seriesis a simple form, in which the orthogonal functions are the complex exponential functions. Web exponential fourier series in [ ]:

Web Exponential Fourier Series A Periodic Signal Is Analyzed In Terms Of Exponential Fourier Series In The Following Three Stages:

Problem suppose f f is a continuous function on interval [−π, π] [ − π, π] such that ∑n∈z|cn| < ∞ ∑ n ∈ z | c n | < ∞. Using (3.17), (3.34a)can thus be. Web the complex and trigonometric forms of fourier series are actually equivalent. Extended keyboard examples upload random.

Compute Answers Using Wolfram's Breakthrough.

Web complex exponential form of fourier series properties of fourier series february 11, 2020 synthesis equation ∞∞ f(t)xx=c0+ckcos(kωot) +dksin(kωot) k=1k=1 2π whereωo=. Web the exponential fourier series is the most widely used form of the fourier series. In this representation, the periodic function x (t) is expressed as a weighted sum. This can be seen with a little algebra.