Cosine In Exponential Form
Cosine In Exponential Form - The sine of the complement of a given angle or arc. As a result, the other hyperbolic functions are meromorphic in the whole complex plane. Web the fourier series can be represented in different forms. Using these formulas, we can. Expz denotes the exponential function. Web the hyperbolic sine and the hyperbolic cosine are entire functions. (in a right triangle) the ratio of the side adjacent to a given angle to the hypotenuse. I am trying to convert a cosine function to its exponential form but i do not know how to do it. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Cosz denotes the complex cosine.
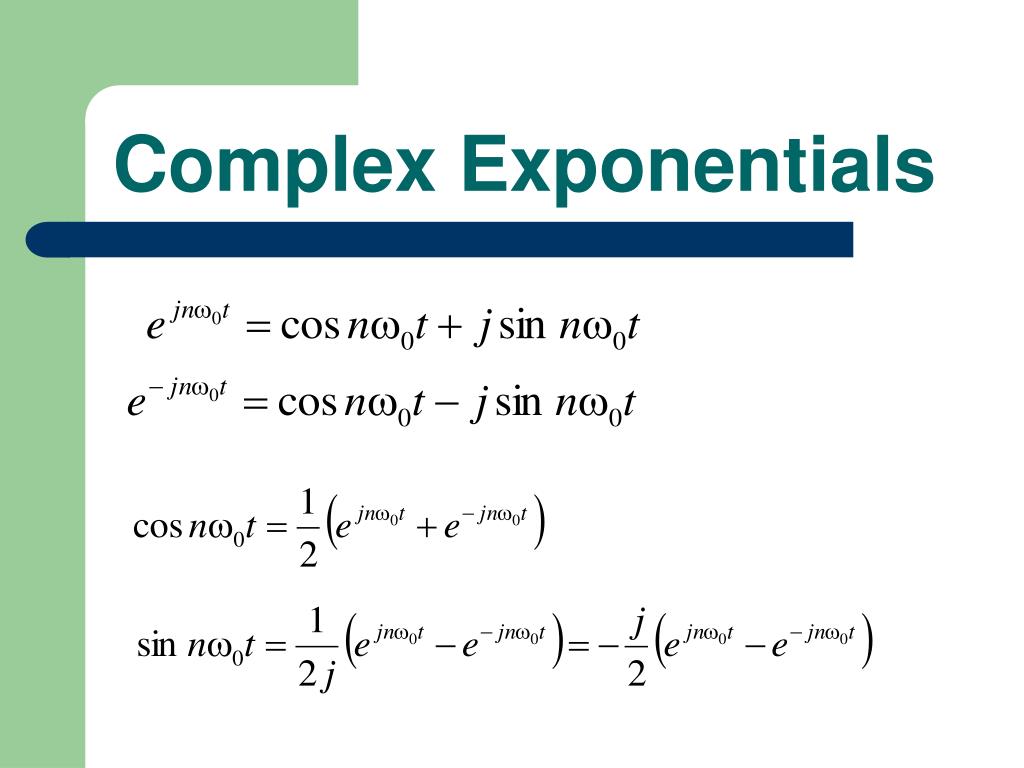
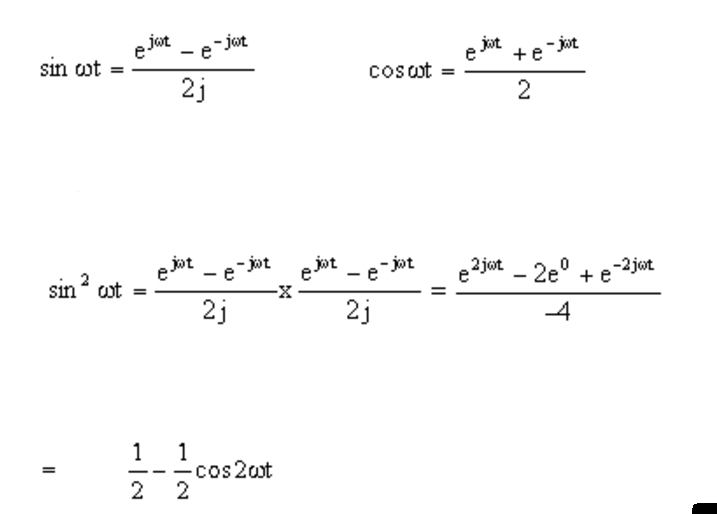
The sine of the complement of a given angle or arc. Cosz = exp(iz) + exp( − iz) 2. A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. I am trying to convert a cosine function to its exponential form but i do not know how to do it. Web the fourier series can be represented in different forms. Web relations between cosine, sine and exponential functions. Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by using the addition formulas for the cosine and sine functions. E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web the hyperbolic sine and the hyperbolic cosine are entire functions. As a result, the other hyperbolic functions are meromorphic in the whole complex plane.
Expz denotes the exponential function. E jx = cos (x) + jsin (x) and the exponential representations of sin & cos, which are derived from euler's formula: Web the hyperbolic sine and the hyperbolic cosine are entire functions. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. For any complex number z ∈ c : Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by using the addition formulas for the cosine and sine functions. Web $\begin{array}{lcl}\cos(2\theta)+i\sin(2\theta) & = & e^{2i\theta} \\ & = & (e^{i \theta})^2 \\ & = & (\cos\theta+i\sin\theta)^2 \\ & = & (\cos\theta)^2+2i\cos θ\sin. Web relations between cosine, sine and exponential functions. Web using the exponential forms of cos(theta) and sin(theta) given in (3.11a, b), prove the following trigonometric identities: Web euler’s formula for complex exponentials according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and.
Exponential cosine fit A phase binned amplitude exemplar (Data) is
Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. As a result, the other hyperbolic functions are meromorphic in the whole complex plane. Web integrals of the form.
Relationship between sine, cosine and exponential function
Web $\begin{array}{lcl}\cos(2\theta)+i\sin(2\theta) & = & e^{2i\theta} \\ & = & (e^{i \theta})^2 \\ & = & (\cos\theta+i\sin\theta)^2 \\ & = & (\cos\theta)^2+2i\cos θ\sin. Web $$e^{ix} = \cos x + i \sin x$$ fwiw, that formula is valid for complex $x$ as well as real $x$. Web the hyperbolic sine and the hyperbolic cosine are entire functions. E jx = cos.
PPT Fourier Series PowerPoint Presentation ID390675
Web integrals of the form z cos(ax)cos(bx)dx; Web relations between cosine, sine and exponential functions. Web the fourier series can be represented in different forms. A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by using the addition formulas for the cosine and sine functions.
Solution One term of a Fourier series in cosine form is 10 cos 40πt
Web $\begin{array}{lcl}\cos(2\theta)+i\sin(2\theta) & = & e^{2i\theta} \\ & = & (e^{i \theta})^2 \\ & = & (\cos\theta+i\sin\theta)^2 \\ & = & (\cos\theta)^2+2i\cos θ\sin. (in a right triangle) the ratio of the side adjacent to a given angle to the hypotenuse. The sine of the complement of a given angle or arc. Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by.
Complex Numbers 4/4 Cos and Sine to Complex Exponential YouTube
Web relations between cosine, sine and exponential functions. Web integrals of the form z cos(ax)cos(bx)dx; The sine of the complement of a given angle or arc. I am trying to convert a cosine function to its exponential form but i do not know how to do it. Andromeda on 10 nov 2021.
EM to Optics 10 Converting Cos & Sine to Complex Exponentials YouTube
The sine of the complement of a given angle or arc. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. Expz denotes the exponential function. (in a right.
Basics of QPSK modulation and display of QPSK signals Electrical
A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. Cosz denotes the complex cosine. Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by using the addition formulas for the cosine and sine functions. For any complex number z ∈ c : Expz denotes the exponential function.
Math Example Cosine Functions in Tabular and Graph Form Example 16
Cosz denotes the complex cosine. Web using the exponential forms of cos(theta) and sin(theta) given in (3.11a, b), prove the following trigonometric identities: Web relations between cosine, sine and exponential functions. For any complex number z ∈ c : Web $$e^{ix} = \cos x + i \sin x$$ fwiw, that formula is valid for complex $x$ as well as real.
Other Math Archive January 29, 2018
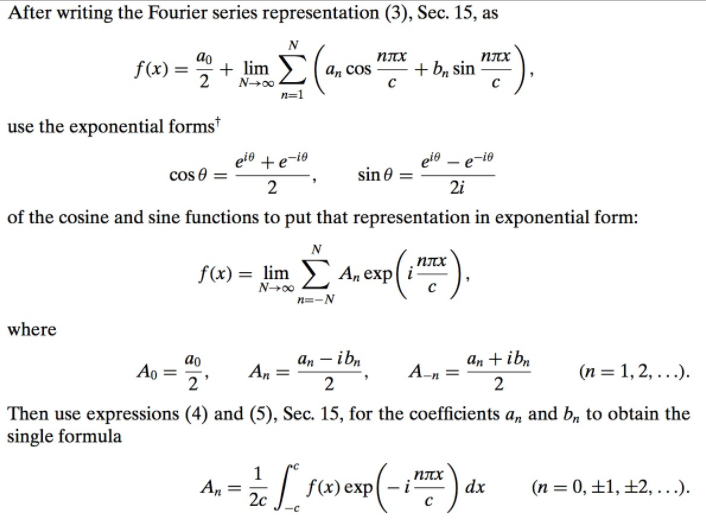
Web the fourier series can be represented in different forms. Web the hyperbolic sine and the hyperbolic cosine are entire functions. Using these formulas, we can. I am trying to convert a cosine function to its exponential form but i do not know how to do it. Web $\begin{array}{lcl}\cos(2\theta)+i\sin(2\theta) & = & e^{2i\theta} \\ & = & (e^{i \theta})^2 \\.
Question Video Converting the Product of Complex Numbers in Polar Form
Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒. For any complex number z ∈ c : Web the fourier series can be represented in different forms. Web.
Web $\Begin{Array}{Lcl}\Cos(2\Theta)+I\Sin(2\Theta) & = & E^{2I\Theta} \\ & = & (E^{I \Theta})^2 \\ & = & (\Cos\Theta+I\Sin\Theta)^2 \\ & = & (\Cos\Theta)^2+2I\Cos Θ\Sin.
Expz denotes the exponential function. (45) (46) (47) from these relations and the properties of exponential multiplication you can painlessly prove all. Web euler’s formula for complex exponentials according to euler, we should regard the complex exponential eit as related to the trigonometric functions cos(t) and. Web the fourier series can be represented in different forms.
E Jx = Cos (X) + Jsin (X) And The Exponential Representations Of Sin & Cos, Which Are Derived From Euler's Formula:
Web integrals of the form z cos(ax)cos(bx)dx; Using these formulas, we can. Web the hyperbolic sine and the hyperbolic cosine are entire functions. Web using the exponential forms of cos(theta) and sin(theta) given in (3.11a, b), prove the following trigonometric identities:
Web $$E^{Ix} = \Cos X + I \Sin X$$ Fwiw, That Formula Is Valid For Complex $X$ As Well As Real $X$.
Z cos(ax)sin(bx)dx or z sin(ax)sin(bx)dx are usually done by using the addition formulas for the cosine and sine functions. A) sin(x + y) = sin(x)cos(y) + cos(x)sin(y) and. As a result, the other hyperbolic functions are meromorphic in the whole complex plane. Cosz denotes the complex cosine.
(In A Right Triangle) The Ratio Of The Side Adjacent To A Given Angle To The Hypotenuse.
Web relations between cosine, sine and exponential functions. Cosz = exp(iz) + exp( − iz) 2. Andromeda on 10 nov 2021. Web we can use euler’s theorem to express sine and cosine in terms of the complex exponential function as s i n c o s 𝜃 = 1 2 𝑖 𝑒 − 𝑒 , 𝜃 = 1 2 𝑒 + 𝑒.